If the Same Force Is Again Applied at T

May 20, 2022 Post a Comment

Physics 121

Final Exam (Solution)

December fifteen, 2005

Instructions: Answer all v questions on these sheets.
Problem I (50 points) is standard multiple-choice, graded only by chosen answer (piece of work needs not be shown).
For Problems II - V (12+12+xvi+x points) bear witness your work, including any equations y'all used, or explicate in words how you arrived at numerical answers.
The exam lasts two hours l minutes.

I. Multiple Choice. Use "Scantron" canvass

0. Please sign the Pledge on your "Scantron" canvass. It applies to the entire exam.

Which of the following is not a vector quantity?
a. temperature
b. velocity
c. acceleration
d. displacement
In one-dimensional motion, the average speed of an object that moves from one place to some other and so back to its original identify has which of the following properties?
a. Information technology is positive.
b. It is negative.
c. It is zero.
d. Information technology tin be positive, negative, or zero.
A chetah tin can run at approximately 30 m/s and a gazelle at 25 m/southward. If both animals are running at full speed, with the gazelle l.0 g ahead, how long before the cheetah catches its casualty?
a. 10 s
b. 25 s
c. thirty s
d. 50 southward
Jeff throws a ball straight up. For which state of affairs is the vertical velocity naught?
a. on the way up
b. at the top
c. on the way back down
d. none of the above
In the example of constant acceleration, the boilerplate velocity equals the instantaneous velocity:
a. at the beginning of the time interval.
b. at the finish of the time interval.
c. one-half-mode through the fourth dimension interval.
d. three-fourths of the way through the time interval.
A rock is thrown straight upwardly with an initial velocity of 19.vi chiliad/due south. What time interval elapses between the rock'due south being thrown and its render to the original launch signal? (Acceleration due to gravity is 9.80 m/s�.)
a. iv.00 s
b. v.00 due south
c. eight.00 s
d. 10.0 s
Human reaction time is normally about 0.20 south. If your lab partner holds a ruler between your finger and thumb and releases it without warning, how far tin you expect the ruler to fall earlier you catch it? The nearest value is:
a. 4.0 cm. c. 16 cm.
b. ix.8 cm. d. twenty cm.
Vector A is three g long and vector B is 4 yard long. The length of the sum of the vectors must be:
a. five m.
b. 7 m.
c. 12 m.
d. some value from 1 g to 7 m.
A aeroplane is moving due northward, straight towards its destination. Its airspeed is 200 mph. A constant breeze is blowing from west to east at forty mph. How long will it take for the plane to travel 200 miles north?
a. one 60 minutes
b. more than one hour
c. less than one hr
d. more information is needed
A 7.0-kg bowling ball experiences a internet force of vii.0 N. What will be its acceleration?
a. 36 m/s�
b. seven.0 yard/s�
c. 5.0 m/s�
d. i.0 m/south�
As I slide a box at constant speed upward a frictionless slope, pulling parallel to the slope, the tension in the rope volition be:
a. greater than the tension would be if the box were stationary.
b. greater than the weight of the box.
c. equal to the weight of the box.
d. less than the weight of the box.
I apply a rope ii.00 m long to swing a 10.0-kg weight around my head with constant athwart velocity, in an exact circumvolve. The tension in the rope is 20.0 Northward. In half a revolution how much work is done by the rope on the weight?
a. 40.0 J
b. 126 J
c. 251 J
d. 0
When an object is dropped from a tower, what is the effect of the air resistance as it falls?
a. does positive work
b. increases the object's kinetic energy
c. increases the object'south potential energy
d. None of the to a higher place choices are valid.
Two blocks are released from the tiptop of a building. Ane falls straight downwardly while the other slides down a shine ramp. If all friction is ignored, which i is moving faster when it reaches the bottom?
a. The cake that went straight downward.
b. The block that went down the ramp.
c. They both will accept the same speed.
d. Insufficient information to work the problem.
A Hooke'due south constabulary leap is compressed a distance d and is used to launch a mass thousand vertically to a meridian h above its starting position. Under double the compression, the spring is now used to launch the mass. How loftier does the mass now rise higher up its starting position?
a. 2 h
b. 1.41 h
c. 3 h
d. iv h
A force of five.0 N is applied to a 20-kg mass on a horizontal frictionless surface. As the speed of the mass increases at a constant acceleration, the power delivered to it by the forcefulness:
a. remains the same.
b. increases.
c. decreases.
d. doubles every 4.0 seconds.
A ball with original momentum +4.0 kg m/s hits a wall and bounces straight back without losing whatever kinetic free energy. The change in momentum of the ball is:
a. 0.
b. -4.0 kg m/s.
c. 8.0 kg chiliad/south.
d. -viii.0 kg grand/s.
A moderate force will intermission an egg. However, an egg dropped on the route unremarkably breaks, while one dropped on the grass usually doesn't suspension. This is because for the egg dropped on the grass:
a. the alter in momentum is greater.
b. the change in momentum is less.
c. the time interval for stopping is greater.
d. the time interval for stopping is less.
A billiard ball collides in an elastic head on collision with a second identical brawl. What is the kinetic free energy of the organisation after the standoff compared to that before collision?
a. the aforementioned as
b. 1 fourth
c. twice
d. four times
Popeye, of mass 70 kg, has just downed a can of spinach. He accelerates apace and stops Bluto, of mass 700 kg (Bluto is very dense), who is charging in at 10 chiliad/southward. What was Popeye's speed?
a. x k/s
b. 31 m/s
c. 50 one thousand/southward
d. 100 g/s
Starting from rest, a bicycle undergoes constant angular acceleration for a period of time T. At which of the following times does the boilerplate angular acceleration equal the instantaneous athwart acceleration?
a. 0.l T
b. 0.67 T
c. 0.71 T
d. all of the to a higher place
A indicate on the rim of a 0.25-m-radius fan blade has centripetal acceleration of 0.20 m/s�. Find the centripetal acceleration of a point 0.05 m from the center of the same cycle.
a. 0.01 m/southward�
b. 0.02 one thousand/south�
c. 0.04 chiliad/south�
d. 0.08 m/s�
When a point on the rim of a 0.30-m-radius bicycle experiences a centripetal acceleration of 4.0 yard/s�, what tangential dispatch does that betoken experience?
a. 1.2 1000/southward�
b. 2.0 m/southward�
c. four.0 thousand/s�
d. Cannot determine with the information given.
Consider a point on a bicycle tire that is momentarily in contact with the ground as the bicycle rolls across the footing with constant speed. The direction for the acceleration for this point at that moment is:
a. upward.
b. downward toward the ground.
c. forward.
d. at that moment the acceleration is zero
2 children seat themselves on a seesaw. The 1 on the left has a weight of 400 Northward while the one on the right weighs 300 N. The fulcrum is at the midpoint of the seesaw. If the child on the left is not at the finish only is 1.50 k from the fulcrum and the seesaw is counterbalanced, what is the torque provided by the weight of the child on the correct? (positive torque is counterclockwise)
a. 600 N�k c. -600 N�m
b. 450 N�m d. -450 N�m
If a net torque is applied to an object, that object will feel:
a. a constant angular speed.
b. an athwart acceleration.
c. a abiding moment of inertia.
d. an increasing moment of inertia.
The World moves about the Sun in an elliptical orbit. Equally the Earth moves closer to the Sunday, which of the following best describes the Earth Sun system's moment of inertia?
a. decreases
b. increases
c. remains constant
d. none of the above choices are valid
A uniform solid sphere rolls down an incline of height 3 m after starting from rest. In guild to summate its speed at the bottom of the incline, i needs to know:
a. the mass of the sphere.
b. the radius of the sphere.
c. the mass and the radius of the sphere.
d. no more than than is given in the trouble.
A solid disk of radius R rolls downwards an incline in time T. The center of the disk is removed up to a radius of R/2. The remaining portion of the disk with its center gone is over again rolled down the same incline. The time information technology takes is:
a. T.
b. more than T.
c. less than T.
d. requires more data than given in the problem to figure out.
The World's gravity exerts no torque on a satellite orbiting the Earth in an elliptical orbit. Compare the motion of the satellite at the indicate nearest the Earth (perigee) to the motion at the signal farthest from the Globe (apogee). At these ii points:
a. the tangential velocities are the same.
b. the angular velocities are the same.
c. the angular momenta are the same.
d. the kinetic energies are the same.
A 15 000-Due north car on a hydraulic lift rests on a cylinder with a piston of radius 0.20 m. If a connecting cylinder with a piston of 0.040-m radius is driven past compressed air, what strength must exist applied to this smaller piston in club to lift the machine?
a. 600 N
b. 1 500 N
c. 3 000 N
d. 15 000 Northward
Equally ice floats in water, about x% of the ice floats above the surface of the water. If we float some ice in a glass of water, what will happen to the water level as the ice melts?
a. The water level will ascent 10% of the volume of the ice that melts.
b. The water level will rise, just not as much every bit the x% indicated in answer A.
c. The water level volition remain unchanged.
d. The water level volition become lower.
A large rock is resting on the lesser of the swimming pool. The normal forcefulness of the bottom of the pool on the stone is equal to the:
a. weight of the rock.
b. weight of the water displaced.
c. sum of the weight of the stone and the weight of the displaced water.
d. difference between the weight of the stone and the weight of the displaced water.
What is the temperature of a organization in thermal equilibrium with some other system fabricated up of ice and water at one atmosphere of pressure?
a. 0�F
b. 273 Yard
c. 0 K
d. 100�C
What happens to a given mass of h2o as it is cooled from 4�C to nothing?
a. expands
b. contracts
c. vaporizes
d. neither expands, contracts, nor vaporizes.
A steel plate has a hole drilled through it. The plate is put into a furnace and heated. What happens to the size of the within diameter of a hole every bit its temperature increases?
a. increases
b. decreases
c. remains abiding
d. becomes elliptical
What happens to a volume of h2o when its temperature is reduced from 8�C to iv�C?
a. density increases
b. density decreases
c. density remains constant
d. vaporizes
What happens to its moment of inertia when a steel deejay is heated?
a. It increases.
b. It decreases.
c. It stays the aforementioned.
d. It increases for one-half the temperature increment and then decreases for the rest of the temperature increase.
An platonic gas is confined to a container with constant volume and the amount of gas is constant. By what factor volition the pressure change if the absolute temperature triples?
a. i/ix
b. 1/3
c. 3.0
d. ix.0
The absolute temperature of an ideal gas is directly proportional to which of the following properties, when taken as an boilerplate, of the molecules of that gas?
a. speed
b. momentum
c. mass
d. kinetic energy
Which of the following statements is true?
a. A hot object contains a lot of estrus.
b. A cold object contains just a footling heat.
c. Objects do not contain rut.
d. Statements a and b are true.
A slice of staff of life contains nigh 100 kcal. If specific heat of a person were 1.00 kcal/kg��C, by how many �C would the temperature of a 70.0kg person increase if all the energy in the breadstuff were converted to estrus?
a. 2.25�C
b. 1.86�C
c. ane.43�C
d. one.00�C
Iced tea is made by adding ice to 1.0 kg of hot tea, initially at ninety�C. How many kg of ice, initially at 0�C, are required to bring the mixture to 10�C? (L_f = fourscore cal/g, c_west = ane cal/gװC)
a. 1.8 kg
b. 1.iv kg
c. 1.0 kg
d. 0.vi kg
In cloud formation, water vapor turns into water droplets which get bigger and bigger until it rains. This will cause the temperature of the air in the clouds to:
a. get warmer.
b. become libation.
c. will not touch on the temperature of the air in the clouds.
d. At that place is no air in clouds.
A system is acted on past its environment in such a way that it receives 50 J of oestrus while simultaneously doing xx J of work. What is its net alter in internal energy?
a. 70 J
b. 30 J
c. zip
d. -30 J
In an isothermal process for an platonic gas system (where the internal energy doesn�t change), which of the following choices best corresponds to the value of the work done on the system?
a. its rut intake
b. twice its heat intake
c. the negative of its oestrus intake
d. twice the negative of its heat intake
The maximum theoretical thermodynamic efficiency of a heat engine operating between hot and common cold reservoirs is a part of which of the following?
a. hot reservoir temperature only
b. cold reservoir temperature simply
c. both hot and cold reservoir temperatures
d. None of the above choices are valid.
Co-ordinate to the second law of thermodynamics, which of the following applies to the heat received from a high temperature reservoir by a rut engine operating in a consummate cycle?
a. must be completely converted to work
b. equals the entropy increase
c. converted completely into internal energy
d. cannot exist completely converted to work
In which organization is heat usually transferred from the cooler part to the warmer office?
a. a stove equally it heats up h2o
b. a refrigerator that is running
c. an electrical fan that is running
d. none of the above, because it is impossible to transfer estrus in this way
A 2.00-kg block of ice is at 0�C and 1 atm while information technology melts completely to water. What is its alter in entropy? (For ice, L_f = 3.34 × 10^five J/kg)
a. zip
b. 584 J/K
c. 1 220 J/K
d. 2 450 J/Yard

Brusque answer questions. Evidence your piece of work!

II. Kinematics

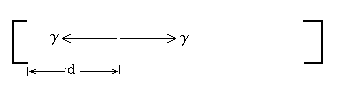Some unproblematic particles tin can be detected simply past what they get out behind when they decay. Such a particle (called π⁰) decays into two particles (called γ's) which move at the speed of light, 3×10^viii m/south, traveling in reverse directions. To discover where the π⁰ was -- that is, the place where the γ's started -- we place 2 γ-counters so that each receives one of the γ's, as in the figure. The distance between the counters is 2 1000, and the left counter responded x^-ix seconds before the right counter. What is the altitude d?

The altitude to i counter is d, that to the other counter is 2 - d (all distances in meters), then the flight time to 1 is d/c, and to the other (2-d)/c, where c is the speed of light. The divergence between these is the given 10^−ninesouthward, then
10⁻⁹ = (ii-d)/c - d/c. Multiply by c: 10⁻⁹ × 3×10⁸ = 0.3 = (ii - d) - d = 2 - 2d, and hence d = �(2 - 0.iii) = 0.85 one thousand
At t = 0 a coach is moving towards its cease on a direct road with a speed 15 m/due south. The autobus cease is 320 yard ahead of the bus, and the bus arrives there at t = twenty s (and then its speed is zero at t = 20 southward). In that 20 s time interval, did the motorcoach
- not accelerate nor decelerate
- only accelerate
- but decelerate
- both advance and decelerate?
Explicate your choice, giving a cogent reason why information technology must be correct.

The bus must certainly decelerate to come to a terminate. Suppose it did not accelerate, then in xx s it could go at virtually (15 m/southward)(20s) = 300m. Only since the cease is further away than that, it must have accelerated earlier decelerating.
Another manner to debate is by computing the 5_av = 320m/20s = 16 m/s. The bus cannot become at an boilerplate speed of 16 g/south by only decelerating from xv m/due south.
The speedometer on a car'southward dashboard reads on a linear scale. The scale 4.5 in long, with speed 0 at 0 cm, and speed 150 km/h = 42 1000/southward at iv.2 in. The car is accelerating at 2 m/s�. What is the speed in inches/2d of the accelerometer "needle" (that is, the pointer indicating the present speed) with respect to the motorcar'south dashboard?

Since it does not matter at what speed the car is going, suppose it starts at speed 0, and accelerates for ane s. Information technology is then going at 2 m/s. The speedometer needle started at speed 0, hence position 0, and reached position 0.2 in after the 1 sec. (Since 42 1000/southward is indicated on the calibration at 4.ii in, ii one thousand/s is indicated at 0.2 in). Hence the needle's speed is 0.two in/ane southward = 0.ii in/south.

III. Dynamics

You are twirling your keys of mass 1 kg on their key string in a vertical circle of radius ten cm = 0.1 m. At the meridian of their circular trajectory the string tension just reaches zip (the keys are in gratuitous fall at that moment).
1. What is the keys' speed at the bottom of the circle?
2. What is their acceleration at the bottom?
3. What is the tension in the cord when the keys are at the bottom?
i. In free fall, gravity provides the centripetal force, and so mg = mv_superlative�/R. (This yields five_top = 1 1000/s if thousand = 10 m/s�, simply it is better not to solve for v at this time because nosotros desire next to use conservation of free energy)
Conservation of energy says (with v = speed at bottom)
�mv� = �mv_top� + mg(2R) = �(mgR) + mg(2R) = (5/2)mgR, then v = √(5gR) = 2.21 m/southward
two. At that place is no horizontal acceleration (angular velocity reaches maximum magnitude at bottom, so momentarily does non change). The etire acceleration is vertical, therefore centripetal, and determined by the speed alone, a = v�/R = 5gR/R = 5g = 49 m/southward�

iii. The centripetal force consists of string tension (up) and gravity (down) so
mv�/R = T − mg T = 5mgR/R + mg = half-dozen mg = 58.8 N
A car (treated as a indicate particle) is moving with constant speed along an S-shaped rail as shown. On the effigy, at the points indicated, accurately draw the car's acceleration vector. Utilize an capricious scale, but keep it the same at all the points.
A roll of newsprint (paper for newspaper) has radius R, and paper is fed from it into the press press with a abiding speed v.
1. What is the curlicue'southward athwart velocity (magnitude as formula, direction description in words)?
2. How much time does it take for ane revolution? (formula in terms of R and 5)
3. If the paper'southward thickness is t, the radius decreases by t in one revolution. What is the angular velocity after this decrease by t? (formula in terms of R, v, t)
4. What is therefore the angular acceleration of the gyre? (slightly complicated formula in terms of R, 5, t)

i. ω = five/R direction along axis of rotation. If newspaper is coming off the lesser of the roll and the paper moves to the right, ω points out at the viewer.

two. Ane revolution moves a length 2πR of paper; to movement that altitude with velocity 5 takes a time Δt = 2πR/v.

iii. Since the radius is now R-t, the angular velocity is ω' = v/(R-t)

iv. α = Δω/Δt = (ω'−ω)/Δt = [⁵/_R-t − ^five/_R] (^v/_2πR) =

5�t

2πR�(R-t)

IV. Fluids

A cart containing a crocodile can roll on a level frictionless rail.
1. Initially the cart and crocodile are at rest, with the croc at the left wall of the cart. The croc at present starts to crawl to the right inside the cart. Volition the cart motility? If and so, in what direction? What law of physics tells you lot?
  The cart will move, in social club to conserve momentum. Frictionless track implies no external force, so the croc's momentum to the right has to be counterbalanced by the cart's momentum to the left.
2. Next the cart is filled with water and starts out once more at remainder, with the croc swimming near the cart's left wall. Then the croc swims to the right. Will the cart move in this instance? Why?
  In this case the cart does not motion. While pond the crocodile displaces and equal weight, and hence equal mass, of water. The water and croc are in the enclosed volume of the cart, so when the croc swims to the right, and equal mass of water moves to the left, and momentum of croc + water is conserved, without the cart having to motion.
  All the same, this assumes that there is no friction between water and cart. If in that location is, there is no reason why the cart should non share in the momentum.
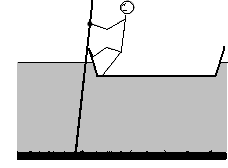Mr and Mrs Johnson (the ones who recently moved) are in a gunkhole, and their motor died. They have no oars, but there is a long rod that will reach to the bottom of the lake. Mr Johnson suggests to stick the rod vertically from the back of the boat into the mud on the lake's bottom, and pull forward on the summit of the rod. Mrs Johnson (as usual thinking most forces) predicts that this won't work, because Mr. Johnson will be pushing astern with his feet as difficult every bit he is pulling forward with his hands. Mr. Johnson (as usual thinking about work) argues "I'll be doing work, that must convert into K.Eastward., hence we and the boat will motion".
1. Volition the boat motility? Explain
  The boat will motion. If it did not, nosotros could use the point of contact between pole and gunkhole as a fixed origin for calculating torques, which would have to add to zero. To balance Mr. Johnson'southward torque, there must be a foce at the bottom terminate of the pole. Only this forcefulness would be an external strength on the boat+pole system, a contradiction to the assumption that the gunkhole (+pole) does not motion.
  To show this without resorting to proof by contradiction, nosotros must take torques about the bottom of the pole, which is certain to exist stock-still. Considering the lever arms of the boat and of Mr Johnson'south hand are unequal, and their torques must exist the same (nosotros tin assume negligible mass, and hence negligible moment of inertia, of the pole), the ii forces must be unequal; their difference will propel the gunkhole.
2. If yeah, think of the gunkhole, occupants, and pole as 1 organisation and fail friction with the water. Where does the force to the system get practical?
  If no, where does the piece of work get that Mr. Johnson does?
  The force is applied at the point where the pole is stuck in the mud of the lake bottom.
  If you answered that the gunkhole does non move, a consistent reply would be that whatever work Mr. Johnson does goes into angle the pole.
A block, of density greater than that of water, is resting on a plane. The coefficient of friction betwixt aeroplane and block is μ = 1. The plane is tilted until the disquisitional angle is reached at which the block begins to slip.
1. What is this critical angle (betwixt plane and horizontal)?
  Since tan θ = μ = one, θ = 45°.
2. The experiment is repeated under h2o. The coefficient of friction remains the same. What is now the critical angle? Explain.
  It is the aforementioned, 45°. The buoyant force is vertical, reverse to the gravitational force. For statics, it is as if the mass of the block were reduced. But the equation for θ is independent of the block's mass.
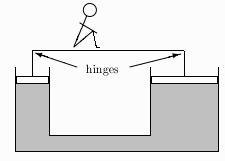Ii cylindrical vessels containing a fluid, one twice the radius of the other, are connected at the lesser, and each carry a piston at the height. The pistons are connected by hinges to a horizontal bar. Where on the bar can a person sit (that is, where can a vertical force be applied to the bar) so the bar does non tilt? (specify what fraction of the bar'south length from left resp. right end)
At equilibrium the pressure on the two pistons is the same, then the force is proportional to the piston's area, four times as large on the right than on the left. So the person should be 4 times further from the left piston than from the right (unlike what is shown in the figure). The person should exist 1/5 of the bar's length from the right end.

V. Lab

Note: The phrase "human error" should non appear in the answer to this question.

John performs an experiment in which he measures the period of a pendulum. He wishes to find the relationship betwixt the period of the pendulum and its length.
Existence a good experimentalist, John measures the time taken for five complete swings, and then obtains the menses by dividing this number by five.

Why does John time five rather than merely one swing?

To reduce the random fault in the measurement, and to increment precision. (The method may nonetheless event in an inaccurate answer if systematic error is involved.)

Later performing the experiment for several lengths, with many trials per length, John creates the following graph:


	The mistake bars are a measure of the distribution of the data collected for each length

John knows that the relationship should be T ~ √Fifty (——), but realizes that his graph likewise admits
a linear fit (· · · · ·).

What does this say almost the accurateness of the experiment?
The average values recorded are very shut to the truthful values, as tin can exist seen by looking at the T ~ √L line. Therefore, the experiment is accurate.
What does this say about the precision of the experiment?
The big error bars indicate that the experiment is not precise.

mckinnonpritur.blogspot.com

Source: https://www.physics.umd.edu/courses/Phys121/Brill/2005/finals.html

McKinnon Pritur

If the Same Force Is Again Applied at T

Post a Comment for "If the Same Force Is Again Applied at T"